Are you ready to explore the fascinating world of group theory and discrete mathematics? If you are, then we have a treat for you! We have gathered some amazing images that showcase the beauty and complexity of these subjects. First up, let's take a look at this stunning image depicting a Hasse diagram. The title of this image is "How to identify lattice in given Hasse diagrams", and it presents a fascinating challenge. The image showcases a complex diagram with multiple nodes and edges, and the goal is to identify the lattice in this diagram. To get started, we need to understand what a lattice is. In the context of group theory, a lattice is a partially ordered set in which any two elements have a unique supremum and a unique infimum. In simpler terms, a lattice is a structure in which each element has a well-defined place in relation to other elements. Now, let's examine the image closely. We can see that there are several elements in this Hasse diagram, and each one is connected to other elements by a series of edges. Our goal is to identify the lattice among these elements. To achieve this, we need to identify a subset of elements which have a unique supremum and a unique infimum. We can see that there are two such subsets in this diagram - a,b,c and d,e,f. In both these subsets, any two elements have a well-defined place in relation to each other, and there is a unique supremum and infimum. Next up, we have another stunning image that showcases the beauty of discrete mathematics. The title of this image is "Lattice of a POSET Relation", and it presents a highly complex diagram that showcases the intricate relationships between different elements. To understand this diagram, we need to first understand what POSET means. A POSET (partially ordered set) is a set equipped with a reflexive, transitive, and antisymmetric binary relation. In simpler terms, it is a set of elements with a well-defined relationship between each element. Now, let's examine the image closely. We can see that there are several elements in this diagram, and each one is connected to other elements by a series of arrows. Our goal is to identify the lattice in this diagram. To achieve this, we need to identify a subset of elements which have a unique supremum and a unique infimum. We can see that there are several such subsets in this diagram - 1,3,6, 2,3,5,6, and 4,5,6. In each of these subsets, any two elements have a well-defined place in relation to each other, and there is a unique supremum and infimum. In conclusion, these images showcase the complexity and beauty of group theory and discrete mathematics. By examining these diagrams closely, we can gain a deeper understanding of the underlying principles and relationships between different elements. So why not take some time to explore these subjects further? You never know what fascinating insights you might discover!
If you are searching about What is a lattice in discrete mathematics? - Quora you've came to the right page. We have 6 Pics about What is a lattice in discrete mathematics? - Quora like discrete mathematics - Lattice of a POSET Relation - Mathematics Stack, group theory - How to identify lattice in given hasse diagrams and also discrete mathematics - Lattice of a POSET Relation - Mathematics Stack. Here it is:
What Is A Lattice In Discrete Mathematics? - Quora
www.quora.com lattice mathematics discrete examples counter
What Is A Lattice In Discrete Mathematics? - Quora
www.quora.com lattice mathematics discrete examples counter
Schematic Of The Discrete Lattices Used In This Study; (a) The D3Q15
www.researchgate.net discrete lattice lattices
Discrete Mathematics Lattices - Javatpoint
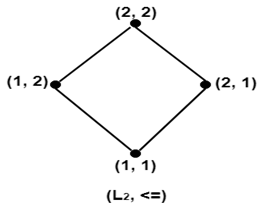www.javatpoint.com discrete mathematics lattices boolean algebra javatpoint lattice topic next
Group Theory - How To Identify Lattice In Given Hasse Diagrams
math.stackexchange.com lattice hasse diagrams given mathematics theory identify math group
Discrete Mathematics - Lattice Of A POSET Relation - Mathematics Stack
math.stackexchange.com poset lattice discrete mathematics math relation partial defined given order
Lattice hasse diagrams given mathematics theory identify math group. Discrete mathematics. Lattice mathematics discrete examples counter
0 Post a Comment: